ST Math is carefully designed by neuroscientists, mathematicians, and educators to use the engaging qualities of games to build a strong conceptual understanding of mathematics. Take a look at some of the elements that make ST Math so powerful.
Problem-Based Learning
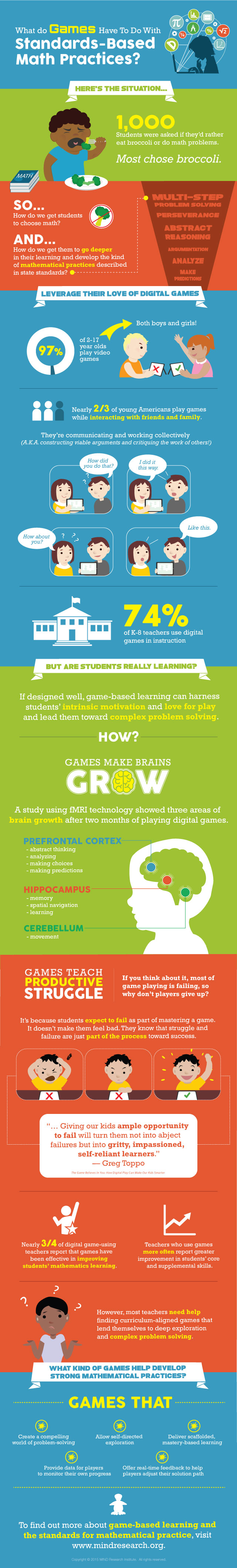
Would you be surprised that students learn better when they figure things out on their own, as compared to being told what to do?
One research study (1) had two very similar groups of young adults solve a math problem. One group received instruction in how to solve it while the other group was told to find their own solution. When the subjects were brought back six days later and presented with a similar problem, the creative group had 10% more correct answers.
More interesting were the results from the functional Magnetic Resonance Imaging (fMRI). Researchers found that the area of the brain involved in working memory was less active in the creative group.
Working memory is that ability to hold things in your mind and is limited in size – usually six to eight bits of information. If you’re solving (2+7) x (8-3), it’s where you hold 9 and 5 before multiplying them.
Why would working memory be taxed more in the algorithm group? They likely didn’t really learn the steps so they were trying to remember how to solve the problem and that took up working memory.
That’s as opposed to the creative group that did what made sense to them. They weren’t trying to recall something that was taught in isolation. They used what they knew.
When students learn in ST Math, they are doing it in the context of what makes sense to them. They are creating their own story about what is going on and figuring it out on their own. That means when they’re presented with similar problems in the future, they’ll have the conceptual understanding to solve it as well as the mindset that they can do it.
(1) Karlsson Wirebring, L., Lithner, J., Jonsson, B., Liljekvist, Y., Norqvist, M., & Lars, N. (2015). Learning mathematics without a suggested solution method : Durable effects on performance and brain activity. Trends in Neuroscience and Education. https://doi.org/10.1016/j.tine.2015.03.002
Learning by Doing (and Failing)
Jack liked math but was reluctant to play ST Math. The teacher had be at his side and reassure him or he wouldn't play. Until one day when he started playing on his own. He was asked about the change . . .
"When I get something wrong in ST Math I don't get in trouble and I don't feel dumb."
As Matthew Peterson, one of the co-founders of MIND Research Institute, said when asked why failure is important,
"From my perspective, it’s about the power of mistakes, that mistakes are one of the best learning vehicles. You can learn more from a mistake, often, than from getting something right. But we fear mistakes.
You get penalized for making mistakes in the normal education system. But in a game you learn tremendously from making mistakes, and you’re not worried as much about making mistakes, unless it’s toward the end of a level or something like that. But from the learning aspect, mistakes are powerful and important and you embrace them."
Learning by doing (and failing) is just one of the benefits of using games in math -- especially if you care about standards.
Informative Feedback
Great computer games take advantage of our natural learning process.
Think for a minute about when you, a friend, a student, or your own children had to learn something. Perhaps it was how to drive, work your new phone, or how to shoot a free throw.
Now think about what it would be like if you pressed a button on your phone and didn’t learn what happened for a day or two. Or if a child shot a basket and found out 20 minutes later whether or not they made it.
The way we learn best in the real world includes immediate and informative feedback.
Press a button on your phone and mail pops up. Shoot a basket and see that it’s a complete air ball. What makes learning happen in the real world is immediate and informative feedback.
When you think about learning a new computer game, the same applies. Let’s say you download and start playing Plants vs. Zombies. Did you read the manual? Is there even an instruction book?
You’re probably going to just start playing and the game is going to gently guide you through learning how to play and what’s important. And as you play, additional plants, zombies, and functionality are introduced and practiced with immediate and informative feedback to make sure you can play successfully.
What’s happening behind the scenes in learning your phone, shooting a basketball, or playing a computer game, is the perception-action cycle which is easiest to talk about using the Learning Cycle.
Take a minute to think about something you’ve learned; maybe how to work a new phone.
Notice – I see an icon on the home screen that looks like an envelope.
Predict – I bet that’s for email.
Analyze – Yep, it wants me to set up my email account.
Connect – So that’s the same as it was on my old phone. I wonder how I send an email?
. . . and the cycle starts again.
Progressive Growth
Researchers at Indiana University found that two out of three students are bored in class every day. Seventy-five percent said it was because the material wasn’t interesting while almost 40 percent said it wasn’t relevant.
The design of ST Math allows students to move at their own pace with low entry points but high ceilings. If the content is easier for a student, they move through the puzzles quickly (although they will be gaining insight and strengthening their conceptual understanding as they go). If they’re having some trouble – we call it good stuck– then it’s clear they’re in the middle of learning. While students are playing the games, they’re constantly making sense of the math for themselves – which cuts down on the boredom.
There’s another problem with progressive growth. It often takes time. And it might take a lot more time for one student than another. As Greg Toppo, K-12 Education Reporter for USA Today and author of The Game Believes in You: How Digital Play Can Make Our Kids Smarter, says
“Your teacher may be overwhelmed, your friends wish you’d finish your homework, and your mom just wants to go to bed. But as JiJi demonstrates, a well-designed game sits and waits . . . and waits. It doesn’t care if that wearisome math problem takes you fifteen seconds or four hours. Do it again. Take all day. The game believes in you.”
Check for Understanding
Think about your answer and then click to reveal our thoughts.
What are the four elements of games that makes it a perfect platform for teaching math?
- Problem-based learning
- Learning by doing (and failing)
- Informative feedback
- Progressive growth
What is the difference between ST Math and games that present routine tasks?
ST Math presents puzzles that engage students in productive struggle and effortful thinking and provides them with immediate, informative feedback.
Why might students prefer to eat broccoli than solve math problems?
They like broccoli.
Math may have been a subject area where they didn't experience success and/or couldn't memorize all the unrelated rules.
Their experience is that math is ________ (boring, hard, stupid, no fun, . . . .)
What is your favorite element in ST Math as a game?
Immediate feedback
Levels get harder
Move at your own pace
Can play it anywhere
. . . .??
Congratulations! You've completed The Essentials course!
